Apoorva Khare’s maths is not just about finding beauty in snowflakes but also has real-world applications like predicting the weather 

Last December, Apoorva Khare was invited to give a talk as part of National Mathematics Day at the Shri Vaishnav Vidyapeeth Vishwavidyalaya in Indore. He was asked to speak to people who were not familiar with advanced mathematics. Apoorva, who is an Associate Professor in the Department of Mathematics, IISc, decided to revisit the first paper he published in his late teens in 1997, in the Furman Electronic Journal of Undergraduate Mathematics, titled “Divisibility Tests.” In the paper, he had found a unified way to test the divisibility of any number.  

Growing up in Bhubaneswar as a son of two physics professors, Apoorva was introduced to the world of maths early in his childhood. Family conversations at home centred on science and books. He grew up around scientists on the Institute of Physics campus, watching them play table tennis. He remembers many of them not just for their academic excellence, but also for their “topspins”. 

“I grew up in a family of academics and became an academic,” he says. More importantly, his father inculcated in him a strong interest in mathematics. As a schoolboy, Apoorva loved working on puzzles. He recalls a simple classic puzzle in which one draws a square, cuts it into halves successively, and counts the number of triangles formed in each step. One can increase the complexity by cutting across the diagonals of the same square using two lines, creating multiple triangles in an ordered ratio.  “Then, you start thinking: ‘Oh, there is a subtlety here, there’s a pattern here,’” he explains.  

Apoorva went on to stand first in Odisha in the Regional Maths Olympiads. He also became fascinated by the works of mathematician Shakuntala Devi. “In one of them, she gave a test for dividing by 19. And that was very intriguing because we have seen tests for 3, 11, and 9 in school, but not 19,” says Apoorva.  

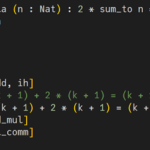
It was his work on this problem that led to the 1997 paper. He picked a number by which to test divisibility. And then found another number whose powers are to be multiplied with the digits of any number (and these products added) to get the test. 

In his own words, he wanted to find the “mystery number” that gave a general divisibility test. Even today, Apoorva is working on uncovering hidden patterns in mathematics. 

Maths in daily life 

Maths, Apoorva says, can be applied not just to find beautiful patterns but also for practical applications. For instance, he works with extensive data given by meteorologists by depicting them as matrices – a systematic way to represent a number that depends on two different things. One could think of ways to represent five commodities and their prices over various years. This is one way to think of a simple version of a matrix.  

However, when the variable depends on many other factors, the number of data points increases, leading to a complex arrangement of numbers in a larger matrix.  

Take the temperature on the Earth. There can be 100,000 variables coming from 100,000 points where temperature can be measured. While dealing with data related to temperature, one needs to ask: “Does the temperature in Siberia depend on the temperature in Delhi?”   

Searching for such dependencies can further expand the matrix to, say, 100,000 rows and 100,000 columns, which even modern computers take time to analyse. “You may need supercomputers or clusters of computers to do meaningful statistical analysis with these,” Apoorva says. 

The temperature at every point depends on the temperature at every other point. “But if I know the temperature across points within a five km radius, can I disregard the rest of the data and still get valid meaning?” Apoorva asks. 

Mathematically, the idea is to make most of the irrelevant correlational values (data points) zero and preserve the meaningful ones as they are. Apoorva’s work involves transforming the data points in a matrix so that the positive property of the matrix is still preserved. This is called thresholding. “If the value is below a certain threshold, then you put that value as zero in the matrix,” Apoorva explains. 

The idea is not just related to predicting temperature – it can be applied to the stock market and even to analyse genetic profiles, in order to predict trends. In the case of the stock market, this involves predicting changes in stock price by looking at larger trends. For genetic profiles, the goal is to look for patterns that hint at the presence of tumours or other diseases by scouring through datasets of gene expressions and health indicators.  

Fighting math-phobia 

Given the largely theoretical nature of his work, Apoorva’s team works on “different islands,” he admits, with a smile. “It is me and one student, and one mathematical problem, and then me, a different student with their problem.”

Having seen and grown up with the beauty of maths, Apoorva is concerned about what he feels is a “math-phobia” among students. According to him, these days, it is almost a fad for some students to say, “I hate maths,” or “I am afraid of it.” He adds: “For some people, even though it is not fashionable to say that they are very bad at English, they think it is okay to say: ‘I am bad at math.’”   

For this reason, at Yale University, where he spent two years pursuing a postdoc, he designed a course on maths and kept it open for people with no background in advanced areas like calculus or trigonometry to attend.  Later, when he moved to Stanford University before joining IISc, Anna Lachowska, another instructor took over the course at Yale. Together with her, Apoorva compiled the course contents into a book titled Beautiful, Simple, Exact, Crazy: Mathematics in the Real World. The motivation behind each chapter was to explain one simple idea and one equation, then work out a lot of abstract examples, and eventually explain real-world applications of concrete mathematical concepts.  

“Mathematics is not something to be afraid of,” Apoorva emphasises.  

Apoorva’s approach to research focuses on deftly juggling smaller goals with one big goal. “Every day you chip away at the big goal, by proving some small step along the way,” he says. “If I can prove something every day, I’m very happy.”